Question

How many grams of silver cholride will be precipitated by adding sufficient silver nitrate to react with 1500.0mL of.400M barium chloride solution?

Answer 1

Answer: 172.2 g of
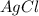`AgCl` is formed by adding sufficient silver nitrate to react with 1500.0mL of 0.400M barium chloride solution.

Step-by-step explanation:

To calculate the moles, we use the equation:

`\text{Number of moles}={\text {Molarty}}* {\text{Volume in L}}=0.400* 1.5L=0.6moles`

`BaCl_2+2AgNO_3\rightarrow 2AgCl+Ba(NO_3)_2`

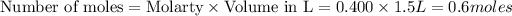
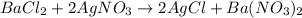
According to stoichiometry:

1 mole of
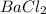`BaCl_2` produce = 2 moles of
`AgCl`

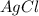
Thus 0.6 moles
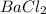`BaCl_2` will produce =
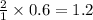`(2)/(1)* 0.6=1.2` moles of
`AgCl`

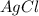
Mass of
`AgCl=moles* {\text {molar mass}}=1.2mol* 143.5g/mol=172.2g`

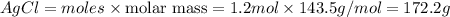
Thus 172.2 g of
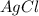`AgCl` is formed by adding sufficient silver nitrate to react with 1500.0mL of 0.400M barium chloride solution.