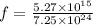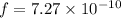Question

Two small aluminum spheres, each of mass 0.0250 kilograms, areseparated by 80.0 centimeters.

How many electrons does each sphere contain? (The atomic mass ofaluminum is 26.982 grams per mole, and its atomic number is13.)

How many electrons would have to be removed from one sphere andadded to the other to cause an attractive force between the spheresof magnitude 1.00*10^{4}N}(roughly one ton)? Assume that the spheres may be treated as pointcharges.

What fraction of all the electrons in one of the spheres does thisrepresent?

Answer 1

Total number of electrons

`N = 7.25 * 10^(24)`

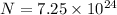
electrons removed from each sphere

`N = 5.27 * 10^(15)`

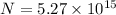
Fraction of electrons transferred is given as

`f = 7.27 * 10^(-10)`

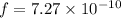
Step-by-step explanation:

As we know that moles is defined as

`n =(mass)/(molar mass)`

`n = (0.0250)/(0.026982)`

`n = 0.93`

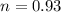
so number of atoms of Al in each sphere is given as

`N = 0.93(6.02 * 10^(23))`

`N = 5.58 * 10^(23)`

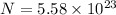
Now number of electrons in each atom is given as

atomic number = number of electrons in each atom = 13

total number of electrons in each sphere is

`N = 13 * (5.58 * 10^(23))`

`N = 7.25 * 10^(24)`

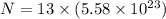
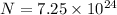
Also we know that force of attraction between them is given as

`F= (kq_1q_2)/(r^2)`

`1.00 * 10^4 = ((9* 10^9)q^2)/(0.80^2)`

`q = 8.4 * 10^(-4) C`

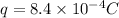
now we have

`q = Ne`

`8.4 * 10^(-4) = N(1.6 * 10^(-19)`

`N = ((8.4 * 10^(-4)))/(1.6 * 10^(-19))`

`N = 5.27 * 10^(15)`

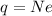
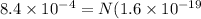
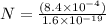
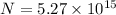
Fraction of electrons transferred is given as

`f = (5.27 * 10^(15))/(7.25 * 10^(24))`

`f = 7.27 * 10^(-10)`