Question

Determine whether the lines l1 and l2 are parallel, skew, or intersecting. If they intersect, find the point of intersection.l1: x=3-2t, y=7+4t, z=-3+8tl2: x=-1-u, y=18+3u, z=7+2u

Answer 1

The lines ared skew

Explanation:

First we check if they are parallel. To do this we compare the ratios of the slopes of each component, and this should be the same:

`(L1_(x) )/(L2_(x) ) =(L1_(y) )/(L2_(y) )=  (L1_(z) )/(L2_(z) )`

`(-2)/(-1) =(4)/(3) =(8)/(2)`

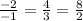
As we can see the ratios are different so they are not parallel lines.

Now we test for intersesction:

we have to equate each component and solve it as a system:

• 3-2t=-1-u
• 7+4t=18+3u
• -3+8t=7+2u

From equation 1: u=2t-4 we replace this in eq. 2

From equation 2: t=1/2 replacing both in equation 3 should give us an identity

From equation 3: we reach 1=3 as this is not true, out hypothesis that the lines intersect is wrong.

Since this lines are not paralel and don't intersect we must conclude that the are skew