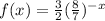Answer:
The exponential decay function is
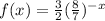 ⇒ 3rd answer
⇒ 3rd answer
Explanation:
* Lets explain the exponential decay function
- The general form of an exponential Function is
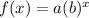 ,
,
where a is the initial value and b is growth factor
- If b > 1 , then the function is exponential growth function
- If 0 < b < 1 , then the function is exponential decay function
- The function
 can be written as
can be written as

# Remember: if 0 < b < 1 , then 1/b > 1 , then change the negative
sign of the power by reciprocal the growth factor to decide the
function is growth or decay
* Lets solve the problem
1.
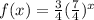
∵ b = 7/4
∵ 7/4 is greater than 1
∴ f(x) is an exponential growth function
2.
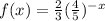
- Change the (-x) to x by reciprocal (4/5) to (5/4)
∴
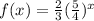
∵ b = 5/4
∵ 5/4 is greater than 1
∴ f(x) is an exponential growth function
3.
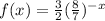
- Change the (-x) to x by reciprocal (8/7) to (7/8)
∴
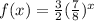
∵ b = 7/8
∵ 7/8 is greater than 0 and smaller than 1 ⇒ 0 < 7/8 < 1
∴ f(x) is an exponential decay function
* The exponential decay function is