Answer:
The angle that the refracted ray in the oil makes with the horizontal is 65.0°.
Step-by-step explanation:
Given that,
Index of refraction of glass = 1.57
Angle = 54°
Index of refraction of oil = 1.40
We need to calculate the angle that the refracted ray, in the oil, makes with the horizontal
Using Snell's law

Put the value into the formula
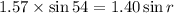
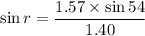
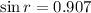
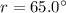
Hence, The angle that the refracted ray in the oil makes with the horizontal is 65.0°.