Question

In a certain state, a survey of 600 workers showed that 35% belonged to a union. Find the 95% confidence interval of true proportion of workers who belong to a union.

Answer 1

The 95% confidence interval of true proportion of workers who belong to a union.

(0.29657 , 0.40343)

Explanation:

Explanation:-

Given sample size 'n' = 600

Given sample proportion p = 35% or 0.35

The 95% confidence interval of true proportion of workers who belong to a union.

`(p^(-) -Z_(0.05) \sqrt{(pq)/(n) } , p^(-) + Z_(0.05) \sqrt{(pq)/(n) } )`

`(0.35 -1.96\sqrt{(0.35 X0.65)/(600) } , 0.35 + 1.96\sqrt{(0.35 X 0.65)/(600) } )`

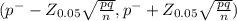
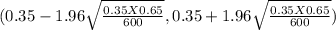
( 0.35 - 1.96 (0.02726) , 0.35 + 1.96 (0.02726)

(0.35 - 0.05343 , 0.35 + 0.05343)

(0.29657 , 0.40343)

Final answer:-

The 95% confidence interval of true proportion of workers who belong to a union.

(0.29657 , 0.40343)