Answer:
Charge,
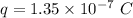
Step-by-step explanation:
It is given that,
Mass of spheres, m = 1 g = 0.001 kg
Distance between spheres, r = 2 cm = 0.02 m
Acceleration when they released,
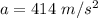
We need to find the magnitude of the charge on each sphere. The electric force is given by :
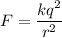
Also, F = ma
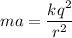
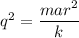
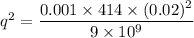
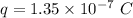
So, the magnitude of charge on each spheres is
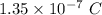 . Hence, this is the required solution.
. Hence, this is the required solution.