Answer:
Length = 18.099 ft
Width = 11.049 ft
Explanation:
let the length of the field be x ft
and the width be y ft
as per the condition given in problem
x=2y-4 -----------(A)
Also the area is given as 200 sqft
Hence
xy=200
Hence from A we get
y(2y-4)=200
taking 2 as GCF out
2y(y-2)=200
Dividing both sides by 2 we get
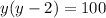

subtracting 100 from both sides

Now we solve the above equation with the help of Quadratic formula which is given in the image attached with this for any equation in form

Here in our case
a=1
b=-1
c=-100
Putting those values in the formula and solving them for y
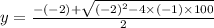
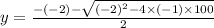
Solving first
![y=\frac{2+√(4+400){2}]()
![y=\frac{2+√(404){2}]()
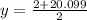
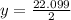
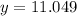
Solving second one
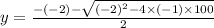
![y=\frac{2-√(4+400){2}]()
![y=\frac{2-√(404){2}]()
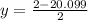
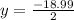
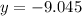
Which is wrong as the width can not be in negative
Our width of the field is
y=11.099
Hence the length will be
x=2y-4
x=2(11.049)-4
x=22.099-4
x=18.099
Hence our length x and width y :
Length = 18.099 ft
Width = 11.049 ft