Answer:
Approximately 0.0898 W/m².
Step-by-step explanation:
The intensity of light measures the power that the light delivers per unit area.
The source in this question delivers a constant power of
 . If the source here is a point source, that
. If the source here is a point source, that
 of power will be spread out evenly over a spherical surface that is centered at the point source. In this case, the radius of the surface will be 9.6 meters.
of power will be spread out evenly over a spherical surface that is centered at the point source. In this case, the radius of the surface will be 9.6 meters.
The surface area of a sphere of radius
 is equal to
is equal to
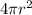 . For the imaginary 9.6-meter sphere here, the surface area will be:
. For the imaginary 9.6-meter sphere here, the surface area will be:
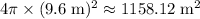 .
.
That
 power is spread out evenly over this 9.6-meter sphere. The power delivered per unit area will be:
power is spread out evenly over this 9.6-meter sphere. The power delivered per unit area will be:
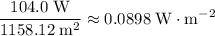 .
.