Answer :
(a) The moles of water produced are 145.35 moles.
(b) The mass of oxygen needed are 3080.8 grams.
Solution for part (a) : Given,
Moles of
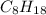 = 16.15 moles
= 16.15 moles
First we have to calculate the moles of
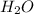
The balanced chemical reaction is,

From the balanced reaction we conclude that
As, 2 moles of
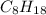 react to give 18 moles of
react to give 18 moles of
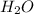
So, 16.15 moles of
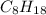 react to give
react to give
 moles of
moles of
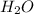
The moles of water produced are 145.35 moles.
Solution for part (b) : Given,
Mass of
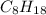 = 878 g
= 878 g
Molar mass of
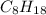 = 114 g/mole
= 114 g/mole
Molar mass of
 = 32 g/mole
= 32 g/mole
First we have to calculate the moles of
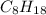 .
.
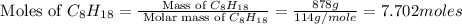
Now we have to calculate the moles of

The balanced chemical reaction is,

From the balanced reaction we conclude that
As, 2 moles of
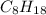 react with 25 moles of
react with 25 moles of

So, 7.702 moles of
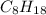 react with
react with
 moles of
moles of

Now we have to calculate the mass of
 .
.
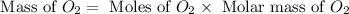
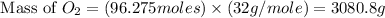
The mass of oxygen needed are 3080.8 grams.