Question

The pressure in a section of horizontal pipe with a diameter of 2.5 cm is 139 kPa. Water ï¬ows through the pipe at 2.9 L/s. If the pressure at a certain point is to be reduced to 101 kPa by constricting a section of the pipe, what should be the diameter of the constricted section? The acceleration of gravity is 9.81 m/s2 . Assume laminar nonviscous ï¬ow.

Answer 1

d = 2*0.87 = 1.75 cm

Step-by-step explanation:

by using flow rate equation to determine the speed in larger pipe

`\phi =\pi r^2 v`

`v = (\phi)/(\pi r^2)`

`= (2900 cm^3/s)/(3.14(1.25cm)^2)`

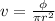
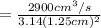
= 591.10 cm/s

= 5.91 m/s

by Bernoulli's EQUATION

`p1 +(1)/(2) \rho v1^2 = p2 +(1)/(2) \rho v2^2`

`139000+ (1)/(2)*1000*5.91^2 = 101000 +(1)/(2)*1000* v2^2`

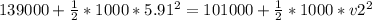
solving for v2

v2 = 10.53 m/s

diameter can be determine by using flow rate equation

`q = v \pi r^2`

`r^2 = (q)/(\pi v)`

`= (2900)/(3.14*1053)`

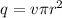
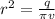
r = 0.87 cm

d = 2*0.87 = 1.75 cm