Answer: The number of moles of ideal gas are 0.0037 moles
Step-by-step explanation:
To calculate the mass of helium gas, we use the equation given by ideal gas:
PV = nRT
where,
P = Pressure of helium gas = 52 cmHg = 520 mm Hg (Conversion factor: 1 cm = 10 mm)
V = Volume of the helium gas = 137 mL = 0.137 L (Conversion factor: 1L = 1000 mL)
n = number of moles of gas = ?
R = Gas constant =
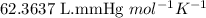
T = Temperature of helium gas =
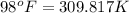 (Conversion factor:
(Conversion factor:
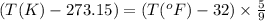 )
)
Putting values in above equation, we get:

Hence, the number of moles of gas is 0.0037 moles.