Question

Find the work by the force F 6i+8j if the object starts from the origin, moves along the x axis to the point (1, 0) then moves in the y direction to the point (1,2). Find the work if instead the object moves diagonally from the origin to the point

Answer 1

Total work done = 22 J

Step-by-step explanation:

F = 6i +8j

This vector represents a constant force which has x component of 6 N and y component of 8 N.

For movement along x axis , work done

force = 6

Displacenent = 1 - 0 = 1 m.

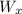`W_x` = force x displacement

= 6 x 1 = 6 J.

In the second lap of movement , there is no movement in x direction so work done in x direction is zero.

Total work done along x direction

= 6 + 0 = 6 J

Now let us calculate work done in y direction.

In the first lap of movement , there is no displacement in y direction ,so work done is zero

In the second lap , displacement in y direction is

2 - 0 = 2 m

Force in y direction is 8 N

So work done

= 8 x 2 = 16 J

Total work done in y direction is

0 + 16 = 16 J

Total work done in the whole process

= 6 + 16 = 22 J.

Total work done = 22 J

Work done is not path dependent so when movement is along the diagnal

work done will be same .