Question

A car rental company offers two plans for renting a car.

Plan A: 20 dollars per day and 8 cents per mile

Plan B: 50 dollars per day with free unlimited mileage

How many miles would you need to drive for plan B to save you money? Show work details.

Answer 1

More than 375 miles.

Explanation:

Let x represent number of miles.

We have been given that according to plan A, the company charges 20 dollars per day and 8 cents per mile.

The cost of driving a car for x miles would be
`0.08x`.

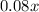The total cost of driving a car for x miles would be
`0.08x+20`.

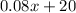To find the number of miles, we can represent our given information in an inequality, where cost of plan A is greater than plan B as:

`0.08x+20>50`

`0.08x+20-20>50-20`

`0.08x>30`

`(0.08x)/(0.08)>(30)/(0.08)`

`x>375`

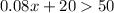
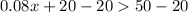
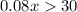
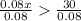
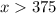
Therefore, we should drive more than 375 miles for plan B to save us money.