Question

A 7.50 kg bowling ball moving 8.39 m/s to the right hits a 1.36 kg bowling pin at rest. Afterwards, the ball moves 6.38 m/s right. What is the final velocity of the pin?

Answer 1

Final speed of the pin is 11.08 m/s.

Step-by-step explanation:

It is given that,

Mass of the bowling ball, m₁ = 7.5 kg

Initial speed of bowling ball, u₁ = 8.39 m/s

Mass of bowling pin, m₂ = 1.36 kg

Initial speed of the bowling pin, u₂ = 0

Final speed of the ball, v₁ = 6.38 m/s

We need to find the final speed (v₂) of the pin. It can be solved using the conservation of linear momentum as :

`m_1u_1+m_2u_2=m_1v_1+m_2v_2`

`m_1u_1+0=m_1v_1+m_2v_2`

`v_2=(m_1u_1-m_1v_1)/(m_2)`

`v_2=(7.5* 8.39-7.5* 6.38)/(1.36)`

`v_2=11.08\ m/s`

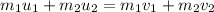
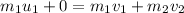
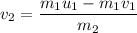
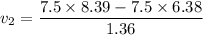
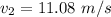
So, the final speed of the pin is 11.08 m/s. Hence, this is the required solution.