Answer:
air velocity at the exit, v' = 15.3 m/s
Given:
Temperature, T =
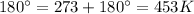
Pressure, P = 300 kPa
Pressure, P' = 100 kPa
isentropic efficiency,
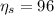
Solution:
In case of isentropic process:
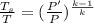
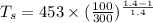
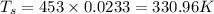
Using steady flow energy eqn:
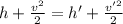
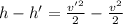
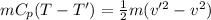
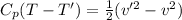
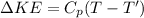 (1)
(1)
Now, by using eqn (1), we can calculate the isentropic kinetic energy:

where
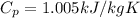
Now,

(a) For actual kinetic energy :
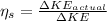
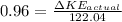
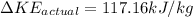
Also,
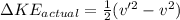 (2)
(2)
Now,
(a) air velocity at the exit, v = 0:
Using eqn (2):
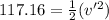
v' = 15.3 m/s