Question

5. If T is a linear transformation such that dim Im(T) = 5 dim Ker(T) = 2 then can we determine dim Dom(T)? Hint: We learned that all these subspaces can be understood as certain subspaces associated to the standard matrix of T.

Answer 1

Yes, we can determine dim Dom(T).

Explanation:

We are given that if T is a linear transformation such that

dim Im(T)=5

Dim Ker(T)=2

We have to tell that we can determine dim Dom(T)

Rank-nullity theorem:

`dim ker(T)+dim Im(T)=Dim dom(T)`

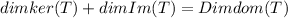
Substitute the values then we get

`5+2=Dim dom (T)`

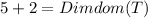
Dim Dom(T)=7

Hence, we can determine dim Dom(T) by using rank- nullity theorem.