Question

Find the fourth point of a parallelogram given the first three (more than one correct answer is possible): (0,6), (5, -1) and (3,-5)

Answer 1

The fourth point of the parallelogram is one point among (-2,2), (2,10) and (8,-12).

Explanation:

Given information: The first three vertices of parallelogram are (0,6), (5, -1) and (3,-5).

Let fourth point of the parallelogram is (a,b).

Diagonals of a parallelogram bisect each other. It means midpoint of both diagonals are same.

Midpoint formula:

`Midpoint=((x_1+x_2)/(2),(y_1+y_2)/(2))`

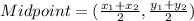
Case 1: If the point (0,6), (5, -1) and (3,-5) are consecutive, then pairs of opposite vertices are (0,6) and (3,-5), (5,-1) and (a,b).

`((0+3)/(2),(6-5)/(2))=((5+a)/(2),(-1+b)/(2))`

`((3)/(2),(1)/(2))=((5+a)/(2),(-1+b)/(2))`

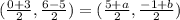
On comparing both sides, we get

`(3)/(2)=(5+a)/(2)`

`3=5+a`

`a=-2`

`(1)/(2)=(-1+b)/(2)`

`1=-1+b`

`b=2`

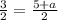
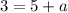
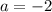
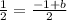
It means the fourth point of the parallelogram is (-2,2).

Case 2: If the point (0,6), (5, -1) and (3,-5) are not consecutive, then pairs of opposite vertices are (0,6) and (5,-1), (3,-5) and (a,b).

`((0+5)/(2),(6-1)/(2))=((3+a)/(2),(-5+b)/(2))`

`((5)/(2),(5)/(2))=((3+a)/(2),(-5+b)/(2))`

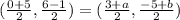
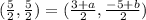
On comparing both sides, we get

`(5)/(2)=(3+a)/(2)`

`5=3+a`

`a=2`

`(5)/(2)=(-5+b)/(2)`

`5=-5+b`

`b=10`

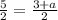
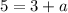
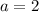
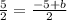
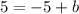
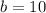
It means the fourth point of the parallelogram is (2,10).

Case 3: If the point (0,6), (5, -1) and (3,-5) are not consecutive, then pairs of opposite vertices are (5,-1) and (3,-5), (0,6) and (a,b).

`((5+3)/(2),(-1-5)/(2))=((0+a)/(2),(6+b)/(2))`

`((8)/(2),(-6)/(2))=((a)/(2),(6+b)/(2))`

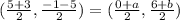
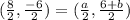
On comparing both sides, we get

`(8)/(2)=(a)/(2)`

`8=a`

`(-6)/(2)=(6+b)/(2)`

`-6=6+b`

`b=-12`

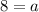
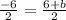
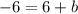
It means the fourth point of the parallelogram is (8,-12).