Question

A ball is thrown straight up and passes point B (at a height of 23.9 m above its starting point O) in 5 s. What was its initial speed? The acceleration of gravity is 9.8 m/s 2 . Answer in units of m/s

Answer 1

The answer is 29.28 m/s

Step-by-step explanation:

This is a classic exercise of projectile motion. If the ball is thrown straight up, then the movement is in one dimension, the y axis, and the equation you need is

`y(t) = y_(0) + v_(0)t - (1)/(2)gt^(2)`

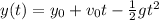
where
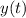`y(t)` is the height at a time "t",
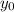`y_(0)` is the initial position,
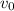`v_(0)` is the initial velocity and "g" is the acceleration of gravity.

If you wonder where this equation came from, you just need to draw the force diagram applied to the ball. Considering that the only force that affects the trajectory of the ball is its weight,

total force = -mg = ma

where "m" and "a" are the mass of the ball and its acceleration respectively, and the negative sign is due to the direction of gravity (straight down). From this equation we get that a = -g, but acceleration is the variation of velocity, then

`(d v)/(d t) = - g`

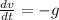
solving the differential equation, we get

`\int\limits^v_{v_(0)} dv = -g\int\limits^t_0dt \\v - v_(0) = -gt \\v(t) = v_(0) - gt`

Once again, velocity is the variation of position, so

`(dy)/(dt) = v_(0)-gt \\ \int\limits^y_{y_(0)} \,dy =\int\limits^t_0 {v_(0) - gt}\,dt \\ y - y_(0) = v_(0)t - (1)/(2)gt^(2)`

then
`y(t) = y_(0) + v_(0)t - (1)/(2)gt^(2)`.

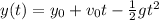Now, getting back to the problem,
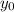`y_(0)` = 0, and
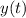`y(t)` = 23.9 m at t = 5 s. So

`23.9 = 0 + v_(0)*5 - (1)/(2)* 9.8 * 5^(2)`, then

`v_(0) = (23.9 + 122.5)/(5) = 29.28 m/s`