Question

For a molecule of O2 at room temperature (300 K), calculate the average angular velocity for rotations about the x or y axes. The distance between the O atoms in the molecule is 0.121 nm.

Answer 1

Answer: 6.65 rad/s

Step-by-step explanation:

Firstly, we need to know that according the kinetic theory of gases, the average kinetic energy
`KE` of a molecule with
`i` degrees of freedom is:

`KE=(i)/(2)kT` (1)

Where:

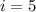`i=5` because oxigen is a diatomic molecule and has 5 degrees of freedom

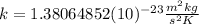`k=1.38064852 (10)^(-23) (m^(2)kg)/(s^(2)K)` is the Boltzman constant

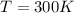`T=300 K` is the temperature

Then:

`KE=(5)/(2)(1.38064852 (10)^(-23) (m^(2)kg)/(s^(2)K))(300 K)` (2)

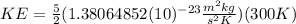`KE=1.035 (10)^(-20) (m^(2)kg)/(s^(2)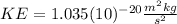)` (3) With this value of average kinetic energy we can find the average angular velocity, with the following equation:

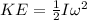`KE=(1)/(2)I \omega^(2)` (4)

Where:

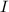`I` is the moment of inertia

`\omega` is the angular velocity

Now,
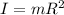`I=m R^(2)` (5)

Being
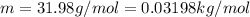`m=31.98 g/mol=0.03198 kg/mol` the molar mass of Oxigen molecule and
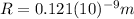`R=0.121(10)^(-9)m` the distance between atoms

`I=(0.03198 kg/mol)(0.121(10)^(-9)m)^(2)` (6)

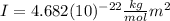`I=4.682(10)^(-22) (kg)/(mol) m^(2)` (7)

Substituting (7) and (3) in (4):

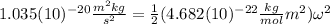`1.035 (10)^(-20) (m^(2)kg)/(s^(2))=(1)/(2) (4.682(10)^(-22) (kg)/(mol) m^(2)) \omega^(2)` (8)

Finding
`\omega`:

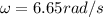`\omega=6.65 rad/s` (9) This is the average angular velocity for a molecule of O2