Question

Find the 64th term of the arithmetic sequence 2, -3, -8,

Answer 1

`a_(64) = -313`

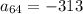
Explanation:
`a_(64) = 2 -135`

This is the Arithmetic Sequence Formula->
`a_(n) = a_(1) + (n+1) d`

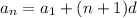
1) The d represents your difference of the equation. You can find it by finding a pattern between the three numbers given, which is -5

2) Now that we've found our difference, we can plug it into this lovely sequence we have
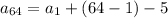`a_(64) = a_(1) + (64-1) -5` (by the way, your n represents your term that you plug into the equation)

3)
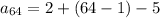`a_(64) = 2 + (64-1) -5` This also means that
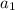`a_(1)` is our first term, which is 2

4) Simplify
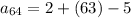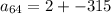
`a_(64) = 2 + (63) -5` -->
`a_(64) = 2 +-315`

5)
`a_(64) = -313`

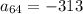
The formula is confusing at first, but it becomes easier once your learn the patterns!