Answer:
The final image of the stamp relative to the diverging lens is 7.01 cm to the left side of the diverging lens.
Step-by-step explanation:
Given that,
Focal length of converging lens = 12.1 cm
Focal length of diverging lens = -5.4 cm
Object distance = 34.5 cm
We need to calculate the image distance for converging lens
Using lens formula
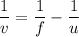
Put the value into the formula
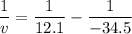
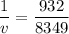
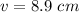
We need to calculate the image distance for diverging lens
Object distance is
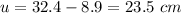
Using lens formula
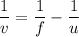
Put the value into the formula
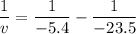
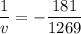
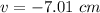
Hence, The final image of the stamp relative to the diverging lens is 7.01 cm to the left side of the diverging lens.