Answer:
The statement is false.
Explanation:
For any vector 'r' we have
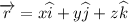
The magnitude is given by
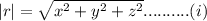
As we know that the upon squaring a term the result is always positive thus the term in right side of equation i is always positive no matter weather the terms (x,y,z) are positive or negative hence we conclude that magnitude of any vectorial quantity is always positive irrespective of it's direction.