Answer:
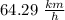
Explanation:
hello
the speed is the relation between a distance traveled and the time used for it and it is given by the equation
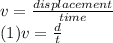
Now, the average speed will be
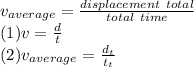
Step 1
I drive 6.0 km at 50 km/h
from (1) it is possible to isolate t
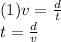
Let
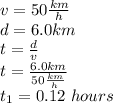
Step 2
then I drive 6.0 km at 90 km/h

Step 3
FInd total time and total displacement
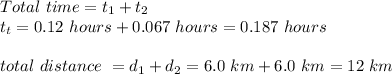
step 4
finally, put these values into the equation(2)

V=64.29 km/h
Have a good day