For this case we have the following inequality:
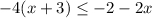
Applying distributive property on the left side we have:

Adding 2x to both sides of the inequality we have:

Adding 12 to both sides of the inequality we have:
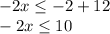
Dividing by 2 to both sides of the inequality:
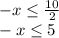
Multiplying by -1 on both sides, taking into account that the sense of inequality changes:
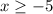
Thus, the solutions are given by all values greater than or equal to -5.
ANswer:
See attached image
Option A