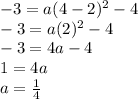Question

Please help asap, thank you!

Answer 1

a =
`(1)/(4)`

Explanation:

Aside from the standard, quadratic form,

`y = ax^2 + bx + c`

Parabolas can also be written as

`y = a(x-h)^2+k`

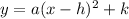
Where a is the scale factor, h represents the distance that the parabola has been translated along the x axis, and k represents the distance the parabola has been shifted up and down the y-axis.

Looking at the table, we see that the function appears to be concave up around x = 2. That means that h in this case is 2. The function is shifted down by -4, because the lowest point on the parabola is (2, -4)

Now we have to solve for a using any points on the table besides x = 2

I will use (4, -3) because it's the easiest option

`- 3 = a(4-2)^2-4\\-3 = a(2)^2-4\\-3 = 4a - 4\\1 = 4a\\a = (1)/(4)`