Answer:
The coordinates of the division point are (0.8 , 1.4)
Explanation:
* Lets explain how to find the point of division
- If point (x , y) divide the line whose endpoints are

and
 at the ratio
at the ratio
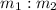 from point
from point
 , then
, then
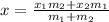 and
and
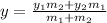
* Lets solve the problem
∵ The endpoint of CD are (-4 , 3) and (8 , -1)
∵ Point (x , y) divides CD directed from C to D at ratio 2 : 3
- By using the rule above
∵ Point (-4 , 3) is

∵ Point (8 , -1) is

∵
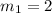 and
and
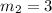
∴
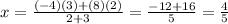
∴
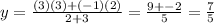
∵ The x-coordinate of the point is 4/5 = 0.8
∵ The y-coordinate of the point is 7/5 = 1.4
∴ The coordinates of the division point are (0.8 , 1.4)