Answer:
0.5357
Explanation:
The waiting times are uniformly distributed between 0 and 7 minutes. We need to find the probability that a randomly selected passenger has a waiting time greater than 3.25 minutes.
The formula to use for uniform distribution is:
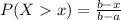
where,
b is the upper limit of the distribution which is 7 in this case.
a is the lower limit of the distribution which is 0 in this case.
x is the concerned value which is 3.25 in this case.
Using these values, we get:

This means, the the probability that a randomly selected passenger has a waiting time greater than 3.25 minutes is 0.5357