Question

There is a famous irrational number called Euler's number, symbolized with an e. Like π , its decimal fo rm never ends or repeats. The first few digits of e are 2.7182818284. A. Between which two square roots of integers could you find this number? _. B. Between which two square roots of integers can you find

Answer 1

`√(7) < e < √(8)=2√(2)`

Answer 2

Explanation:

Given that in Mathematics there is a famous irrational number called Euler's number, symbolized with an e.

This number lies between 2 and 3 and have approximate value as

2.7182818281

Since this lies between 2 and 3, the required integers would be between 4 and 9

Let us find square root of all integers from 4 to 9

`√(4) =2\\√(5) =2.236\\√(6) =2.449\\√(8) =2.828`

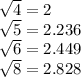
Thus this irrational numbers lies between square root of 7 and square root of 8.