Answer:
9 cm.
Step-by-step explanation:
The energy used for stretch the spring from
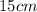 to
to
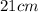 will be ,
will be ,
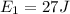
The energy used for stretch the spring from
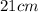 to
to
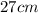 will be ,
will be ,

using the energy of spring formula ,we find that
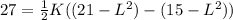
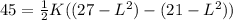
Dividing both the equation will get,
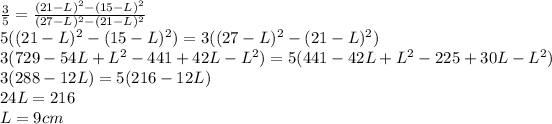
Therefore, the natural length of the spring is, 9 cm.