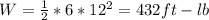Question

If the work required to stretch a spring 2 ft beyond its natural length is 12 ft-lb, how much work is needed to stretch it 12 in. beyond its natural length?

Answer 1

The work required to stretch a spring 12 ft beyond its natural length is 432 ft-lb

Step-by-step explanation:

The work to stretch a spring is calculated using the formula:

`W = (1)/(2)kx^(2)` Equation (1)

W = work in ft-lb

k = spring constant in lb/ft

x = spring deformation in ft

we clear k from the equation (1)

`k = (2W)/(x^(2))` Equation (2)

We replace x = 2ft, W = 12 ft-lb in the equation (2)

`k = (2*12)/(2^(2)) = 6 (lb)/(ft)`

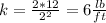
Calculation of work required to stretch spring 12 ft

We replace k = 6 lb/ft and x = 12ft in the equation (1)

`W = (1)/(2) * 6 * 12^(2) = 432 ft-lb`