Answer:
The statement is true
Explanation:
The definition of complement rule states that the sum of the probabilities of an event occurring and not occurring is equal to 1. From there we can obtain the probability of an event occurring to verify the given statement of the question.
Complement Rule.
Let
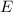 be an event that is occurring and let be
be an event that is occurring and let be
 the complement, that is the event not happening, the complement rule is defined as:
the complement, that is the event not happening, the complement rule is defined as:
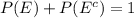
The sum of the probabilities of an event occurring and not occurring is equal to 1.
Verifying the given statement.
In order to verify the given statement, we can solve for the probability of the event occurring that will give us
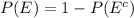
Thus we get that the probability of an event occurring is the subtraction of 1 - the probability of the event not occurring, which makes the given statement true