Question

The junior and senior class officers are sponsoring a homecoming dance. The total cost for the facilities and catering is $45 per person plus a $2500 deposit. Write and graph an equation to represent the average cost per person. Then explain any limitations to the domain and range.

Answer 1

Domain is (0,∞)

Range is (45, ∞)

Explanation:

Let x represents the number of persons and f(x) represents the average cost per person,

∵ Fixed cost = $ 2500

Also, additional cost is $ 45 per person,

So, the total cost for x persons ( in dollars ) = fixed deposit + additional cost for x persons,

= 2500 + 45x

`\text{Average cost for x persons}=\frac{\text{Total cost}}{x}`

`\implies f(x)=(2500+45x)/(x)`

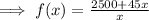
Which is a rational function,

∵ A rational function is defined for all real numbers except those for denominator = 0

Denominator of f(x) = x

So, f(x) is defined for all real numbers except x ≠ 0,

Now, the cost can not be negative,

⇒ x > 0

Hence, the domain for f(x) is (0, ∞)

Again,

`f(x)=45+(2500)/(x)`

If
`x\rightarrow \infty`

`f(x)\rightarrow 45`

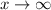
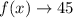
So, 45 < f(x) < ∞

i.e., range of f(x) is (45, ∞)