Question

AB has coordinates A(-5,9) and B(7,- 7). Points P, Q, and I are collinear

points in AB with coordinates P(-2,5), Q(1, 1), and T(4, -3).
Part A: Which of the following line segments would contain the point that
partitions AB into a ratio of 3: 2?

Answer 1

`\overline{QT}`

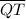
Explanation:

We want to find the coordinates of a certain point C(x,y) such that C divides
`A(x_1,y_1)` and
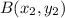`B(x_2,y_2)` in the ratio m:n=3:2

The x-coordinate is given by:

`x=(mx_2+nx_1)/(m+n)`

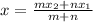
The y-coordinate is given by:

`y=(my_2+ny_1)/(m+n)`

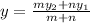
AB has coordinates A(-5,9) and B(7,- 7)

We substitute the values to get:

`x=(3*7+2*-5)/(3+2)`

`x=(21-10)/(5)`

`x=(11)/(5)`

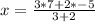
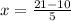
and

`y=(3*-7+2*9)/(3+2)`

`y=(-21+18)/(5)`

`y=-(3)/(5)`

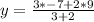
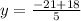
Therefore C has coordinates
`((11)/(5),-(3)/(5))`

The line segment that contains C is
`\overline{QT}`

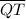
See attachment.