Answer:
8th year
Explanation:
The yearly income or loss of the investment is modeled by a quadratic function. The maximum or minimum value of the quadratic function occurs at its vertex. Since, the coefficient of the squared term is negative, the given function will have a maximum value at its vertex.
The vertex of a quadratic function occurs at =
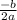
Here,
a = coefficient of squared term = -0.1
b = coefficient of linear term = 1.6
Using these values, we get:
Vertex occurs at = t =
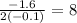
This means, the real estate investment will reach its maximum in 8th year.