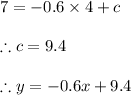Answer:
The equation of line is
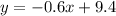
Explanation:
Since the line is perpendicular to the line passing through (-1,-2) and (5,8) the product of it's slope and the line is related as
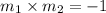
Slope of line through (-1,-2) and (5,8) is
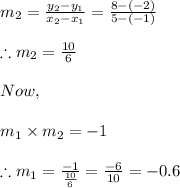
Now we know that general equation of line is given by
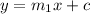
Applying value we get
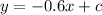
The value of 'c' can be obtained as we know that the line is passing through (4,7)
Thus we have