Question

Which of the following is the correct graph of the solution to the inequality −18 > −5x + 2 ≥ −48?

(A) number line with a closed circle on 4 with shading to the left and an open circle on 10 with shading to the right

(B) number line with an open circle on 4 with shading to the left and a closed circle on 10 with shading to the right

(C) number line with a closed circle on 4 with shading to the right and an open circle on 10 with shading to the left

(D)number line with an open circle on 4 with shading to the right and a closed circle on 10 with shading to the left

Answer 1

D

Explanation:

Answer 2

Option D. A number line with an open circle on 4 with shading to the right and a closed circle on 10 with shading to the left

Explanation:

we have

`-18 > -5x+2 \geq -48`

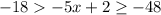
Divide the compound inequality into two inequalities

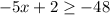`-5x+2 \geq -48` -----> inequality A

`-5x \geq -48-2`

`-5x \geq -50`

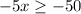
Multiply by -1 both sides

`5x \leq 50`

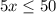
Divide by 5 both sides

`x \leq 10`

The solution of inequality A is the interval ----> (-∞,10]

All real numbers less than or equal to 10

`-18 > -5x+2` -----> inequality B

`-18-2 > -5x`

`-20 > -5x`

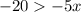
Multiply by -1 both sides

`20 < 5x`

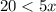
Divide by 5 both sides

`4 < x`

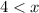
Rewrite

`x > 4`

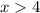
The solution of the inequality B is the interval ----> (4,∞)

All real numbers greater than 4

The solution of the compound inequality is

(-∞,10] ∩ (4,∞)= (4,10]

All real numbers greater than 4 and less than or equal to 10

A number line with an open circle on 4 with shading to the right and a closed circle on 10 with shading to the left