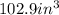Question

a sphere with a diameter 6 inches is inscribed in a cube. WHat is the volume of the space between the sphere and the cube?

Answer 1

`102.9in^(3)`

Explanation:

In order to calculate the space between the sphere and the cube we need to calculate the volume of the cube and then the volume of the sphere. I have attached an illustration below to help you better understand the situation.

`Volume.Cube = a^(3)`

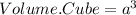
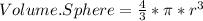`Volume.Sphere = (4)/(3)*\pi  *r^(3)` .... r is radius which is half of diameter.

Now that we know the formulas we can solve for the volumes.

`Volume.Cube = 6^(3)`

`Volume.Cube = 216.in^(3)`

`Volume.Sphere = (4)/(3)*\pi  *r^(3)`

`Volume.Sphere = 113.097in^(3)`

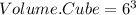
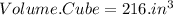
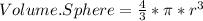
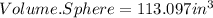
Now we can subtract the volume of the sphere from the volume of the cube in order to calculate the space between them

`216-113.1 = 102.9in^(3)`

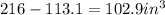
The space between is that of
`102.9in^(3)`