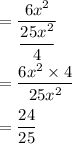Question

The perimeters of square region S and rectangular region R are equal. If the sides of R are in the ratio 2 : 3, what is the ratio of the area of region R to the area of region S ?

Answer 1

Answer with Step-by-step explanation:

the sides of rectangular region R are in the ratio 2 : 3.

i.e. If length=2x

then, breath=3x

Perimeter of rectangular region=2(length+breath)

= 2(2x+3x)

=10x

and Area of rectangular region=2x×3x

=length×breath

=6x²

Let s be the side length of square region S

Perimeter of square region=4s

The perimeters of square region S and rectangular region R are equal.

i.e. 4s=10x

s=
`(5)/(2)x`

Area of square region=s²

=
`(5)/(2)x* (5)/(2)x`

=
`(25)/(4)x^2`

Ratio of the area of region R to the area of region S

`=6x^2:(25)/(4)x^2 \\\\=6:(25)/(4)\\\\=24:25`

Hence, the ratio of the area of region R to the area of region S is:

24:25

Answer 2

The ratio of the area of region R to the area of region S is:

`(24)/(25)`

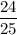
Explanation:

The sides of R are in the ratio : 2:3

Let the length of R be: 2x

and the width of R be: 3x

i.e. The perimeter of R is given by:

`Perimeter\ of\ R=2(2x+3x)`

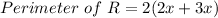
( Since, the perimeter of a rectangle with length L and breadth or width B is given by:

`Perimeter=2(L+B)` )

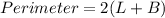Hence, we get:

`Perimeter\ of\ R=2(5x)`

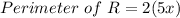
i.e.

`Perimeter\ of\ R=10x`

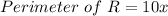
Also, let " s " denote the side of the square region.

We know that the perimeter of a square with side " s " is given by:

`\text{Perimeter\ of\ square}=4s`

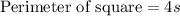
Now, it is given that:

The perimeters of square region S and rectangular region R are equal.

i.e.

`4s=10x\\\\i.e.\\\\s=(10x)/(4)\\\\s=(5x)/(2)`

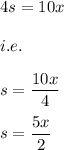
Now, we know that the area of a square is given by:

`\text{Area\ of\ square}=s^2`

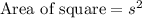
and

`\text{Area\ of\ Rectangle}=L* B`

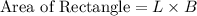
Hence, we get:

`\text{Area\ of\ square}=((5x)/(2))^2=(25x^2)/(4)`

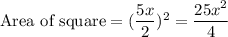
and

`\text{Area\ of\ Rectangle}=2x* 3x`

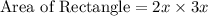
i.e.

`\text{Area\ of\ Rectangle}=6x^2`

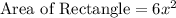
Hence,

Ratio of the area of region R to the area of region S is:

`=(6x^2)/((25x^2)/(4))\\\\=(6x^2* 4)/(25x^2)\\\\=(24)/(25)`