Answer:
23.275 years
Step-by-step explanation:
Given:
Present value or the amount invested = $ 300,000
Rate = 6% compounded quarterly
i.e the rate of return quarterly will be = 6% / 4 = 1.5%
Quarterly payment = $ 6,000
Annuity = $ 0
Now,
Present value of annuity = Part payment × (
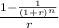 )
)
where,
n is the number of quarters
on substituting the values in the formula, we get
$ 300,000 = $ 6000 × (
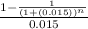 )
)
or
50 × 0.015 = (
 )
)
or
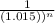 = 1 - 0.75
= 1 - 0.75
or
4 =
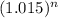
taking log both the sides
we get
log 4 = n × log 1.015
or
0.602 = n × 0.0064
or
n = 93.101 quarters
or
n = 93.101 / 4 = 23.275 years