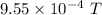Answer:
The potential difference and the magnetic field are 200.655 V and
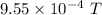
Step-by-step explanation:
Given that,
Speed

radius = 5.0 cm
We need to calculate the potential difference
We know that,
The kinetic energy acquired by electron
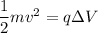
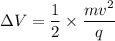
Where, m = mass of electron
q = charge
v = velocity
Put the value into the formula
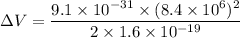
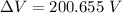
(b). We need to calculate the magnetic field
Using formula of magnetic field
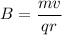
Put the value into the formula
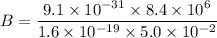
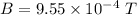
Hence, The potential difference and the magnetic field are 200.655 V and