Answer:
The coordinates of the rest stops are (-1 , -1/3) and (1 , 4/3)
Explanation:
* Lets explain how to solve the problem
- The rest spots between the two towns will divide the highway
into three equal parts
- Each part of the highway will be 1/3 the distance between the two
towns, so the first rest stop will divide the highway at ratio 1 : 2 and
the second rest stop will be the midway between the first rest stop
and the second town
- The location of the first town is (-3 , -2) and the location of the
second town is (3 , 3)
- Assume that the location of the first rest stop is (x , y)
- If point (x , y) divide a line whose endpoints are

and
 at ratio
at ratio
 , then
, then
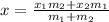 , and
, and
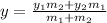
- Let (-3 , -2) is
 and (3 , 3) is
and (3 , 3) is

and
 = 1 : 2
= 1 : 2
∴
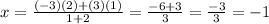
∴
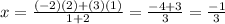
∴ The location of the first rest stop is (-1 , -1/3)
∵ The second rest stop is the midway between the first rest stop
and the second town
∴ The second stop location M is the mid-point between (x , y) and

- The mid-point M is
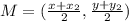
∴
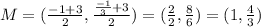
∴ The location of the second rest stop is (1 , 4/3)
* The coordinates of the rest stops are (-1 , -1/3) and (1 , 4/3)