Question

A Styrofoam ice box has a total area of 0.950 m2and walls with an average thickness of 2.50 cm. The box contains ice, water, and canned beverages at 0ºC . The inside of the box is kept cold by melting ice. How much ice melts in one day if the ice box is kept in the trunk of a car at35.0ºC ?

Answer 1

The mass of the ice is 3.44 kg.

Step-by-step explanation:

Given that,

Area of ice box A= 0.950 m²

Thickness d= 2.50 cm

Temperature T₁= 0° C

Temperature T₂= 35.0°C

Time t = 1 day = 86400 s

We need to calculate the mass of ice

Using equation of rat of heat transfer by conduction

`(Q)/(t)=(kA(T_(2)-T_(1)))/(d)`

`(Q)/(t)=(0.010*0.950(35-0))/(2.50*10^(-2))`

`(Q)/(t)=13.3\ j/s`

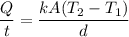
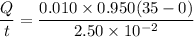
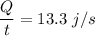
The heat is used to melt the ice

`Q=mL_(f)`

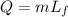
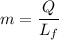`m=(Q)/(L_(f))`....(I)

We need to calculate the heat

`Q = (Q)/(t)* t`

`Q=13.3*86400`

`Q=1149120=1.15*10^(6)\ J`

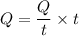
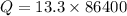
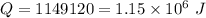
Put the value of Q in the equation (I)

`m=(1.15*10^(6))/(334*10^(3))`

`m=3.44\ kg`

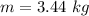
Hence, The mass of the ice is 3.44 kg.