Question

A pair of closely spaced slits is illuminated with 650.0-nm light in a Young's double-slit experiment. During the experiment, one of the two slits is covered by an ultrathin Lucite plate with index of refraction n = 1.485. What is the minimum thickness of the Lucite plate that produces a dark fringe at the center of the viewing screen?

Answer 1

Thickness = 670.10 nm

Explanation:

Given that:

The refractive index of ultrathin Lucite plate = 1.485

The wavelength of the light = 650 nm

The minimum thickness that produces a dark fringe at the center can be calculated by using the formula shown below as:

`Thickness=\frac {\lambda}{2* (n-1)}`

Where, n is the refractive index of ultrathin Lucite plate = 1.485

`{\lambda}` is the wavelength

So, thickness is:

`Thickness=\frac {650\ nm}{2* (1.485-1)}`

Thickness = 670.10 nm

Answer 2

The minimum thickness of the Lucite plate is 0.670 μm.

Step-by-step explanation:

Given that,

Wavelength = 650.0 nm

Index of refraction = 1.485

We need to calculate the minimum thickness of the Lucite plate

Using formula of thickness

`t(n-1)=(\lambda)/(2)`

`t=(\lambda)/(2(n-1))`

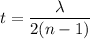
Where, n = Index of refraction

`\lambda` = wavelength

Put the value into the formula

`t=(650.0*10^(-9))/(2(1.485-1))`

`t =0.670\ \mu m`

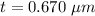
Hence, The minimum thickness of the Lucite plate is 0.670 μm.