Question

Suppose that grades on a midterm and a final have a correlation coefficient of .5 and both exams have an average score of 75 and a standard deviation of 10. a. Ifastudent’sscoreonthemidtermis95,whatwouldyoupredicthisscoreon the final to be?

Answer 1

85

Explanation:

The correlation coefficient relates the standarized values of the midterms a final score in this way:

`(y - y^)/(sy)  = r* (x - x^)/(sx)`

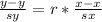
where y is the final score that needs to be found, y^ is the average score on the finals, sy is the standar deviation for the scores on the finals, x is the midterm score, x^ is the average score on the midterms and sx y the standard deviation of the scores on the midterms.

If we issolate y on the equation and solve with the data we have, we get that:

r* \frac{x - x^}{sx}[/tex] = 0.5 *
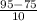`(95 - 75)/(10)` = 1

y = 75 + 1*10 = 85

This means the predicted final score will be 85.