Question

The pressure inside a champagne bottle can be quite high and can launch a cork explosively. Suppose you open a bottle at sea level. The absolute pressure inside a champagne bottle is 6 times atmospheric pressure; the cork has a mass of 7.5 g and a diameter of 18 mm.Assume that once the cork starts to move, the only force that matters is the pressure force.

What is the acceleration of the cork? Express your answer with the appropriate units.

Answer 1

The acceleration of the cork is 17189.33 m/s².

Step-by-step explanation:

Given that,

Mass of cork = 7.5 g

Diameter = 18 mm

The absolute pressure inside a champagne bottle is 6 times atmospheric pressure.

We need to calculate the radius of cork

Using formula of radius

`r=(D)/(2)`

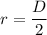
Put the value into the formula

`r=(18)/(2)`

`r=9\ mm`

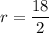
We need to calculate the force

Using formula of force

`F=\Delta P* A`

`F=\Delta P(\pi r^2)`

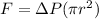
Put the value into the formula

`F=(6-1)*(1.01325*10^(5))*\pi*(9*10^(-3))^2`

`F=128.92\ N`

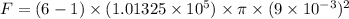
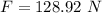
We need to calculate the acceleration of the cork

Using formula of acceleration

`F=ma`

`a=(F)/(m)`

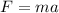
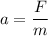
Put the value into the formula

`a=(128.92)/(7.5*10^(-3))`

`a=17189.33\ m/s^2`

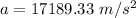
Hence, The acceleration of the cork is 17189.33 m/s².

Answer 2

17 km/s² approx

Step-by-step explanation:

Excess pressure from inside

= 5 times atmospheric pressure

= 5 x 10⁵ Nm⁻²

Total force on the cork = pressure x area

=5 x 10⁵ x 3.14 x 9² x 10⁻⁶ N = 127.17 N

Acceleration of cork = force / mass

`(127.17)/(7.5*10^(-3))`

= 16956 m s⁻².