Question

The lengths, in inches, of adult corn snakes are normally distributed with a population standard deviation of 6 inches and an unknown population mean. If a random sample of 18 snakes is taken and results in a sample mean of 61 inches, find the margin of error (ME) of the confidence interval with a 90% confidence level. Round your answer to three decimal places.

Answer 1

Answer: 2.460

Explanation:

The formula of Margin of Error for (n<30):-

`ME=t_(\alpha/2)(\sigma)/(√(n))`

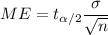
Given : Sample size : n= 18

Level of confidence = 0.90

Significance level :
`\alpha=1-0.90=0.1`

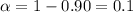
Using the t-distribution table ,

Critical value :
`t_(n-1, \alpha/2)=t_(17,0.05)= 1.7396`

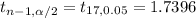
Standard deviation:
`\sigma=\text{ 6 inches }`

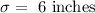
Then, we have

`ME=( 1.7396)(6)/(√(18))\approx2.460`

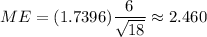
Hence, the margin of error (ME) of the confidence interval with a 90% confidence level = 2.460