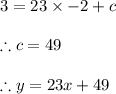Answer:
The equation of the line is
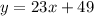
Explanation:
The general equation of a line is given by
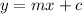
Where,
'm' is the slope of the line
'c' is the intercept of the line
Now we are given that
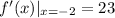
By definition the derivative of a function at any point is the slope of the tangent at that point
Thus the slope of the line passing x = -2 is 23
Hence 'm' = 23
Thus the equation of the line becomes
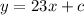
to find the intercept we are given that the line passes through (-2,3)
Using this information in the above equation of line we get