Answer:
Given,
S is the between R and T on line segment RT.
Thus, RS + ST = RT,
RS and ST.
a. If RS = 2x-10. ST = x-4. and RT = 21,
2x - 10 + x - 4 = 21
3x - 14 = 21
3x = 35

RS =
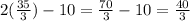
ST =
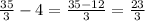
b. RS = 3x-16. ST = 4x-8. and RT= 60,
3x - 16 + 4x - 8 = 60
7x - 24 = 60
7x = 84
 = 12,
= 12,
RS = 3(12) - 16 = 36 - 16 = 20,
ST = 4(12) - 16 = 48 - 16 = 32
c. RS= 2x-8. ST = 3x-10. and RT = 17.
2x - 8 + 3x - 10 = 17
5x - 18 = 17
5x = 35
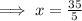 = 7,
= 7,
RS = 2(7) - 8 = 14 - 8 = 6,
ST = 3(7) - 10 = 21 - 10 = 11