Question

Determine whether each pair of functions are inverse functions. 1) f(x) = 8x – 10, g(x) = (x + 10) 2) f(x) = 4x + 5, g(x) = 4x – 5

Answer 1

Explanation:

To find the inverse of a function you take it in y=f(x) form, switch x and y and then solve for the new y.

So I'll do the first one.

f(x) = 8x-10

y=8x-10 Now switch x and y

x = 8y-10 Now solve for y.

x+10=8y

(x+10)/8 = y

g(x) is not that, so it is not the inverse. Can you figure out the second one?

Answer 2

Both are not inverse functions.

Explanation:

To find : Determine whether each pair of functions are inverse functions ?

Solution :

To determine the functions has to satisfy the condition,

`f(g(x))=x=g(f(x))`

1)
`f(x) = 8x-10,\  g(x) = (x + 10)`

`f(g(x))=f(x+10)`

`f(g(x))=8(x+10)-10`

`f(g(x))=8x+80-10`

`f(g(x))=8x+70`

As
`f(g(x))\\eq x`

`g(f(x))=g(8x-10)`

`g(f(x))=8x-10+10`

`g(f(x))=8x`

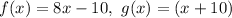
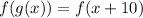
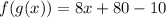
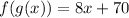
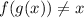
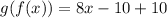
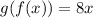
As the condition is not satisfied.

2)
`f(x) =4x+5,\  g(x) =4x-5`

`f(g(x))=f(4x-5)`

`f(g(x))=4(4x-5)+5`

`f(g(x))=16x-20+5`

`f(g(x))=16x-15`

As
`f(g(x))\\eq x`

`g(f(x))=g(4x+5)`

`g(f(x))=4(4x+5)-5`

`g(f(x))=16x+20-5`

`g(f(x))=16x+15`

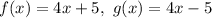
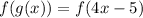
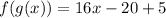
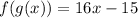
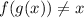
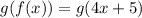
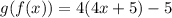
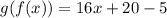
As the condition is not satisfied.

Both are not inverse functions.